poj 2447 RSA

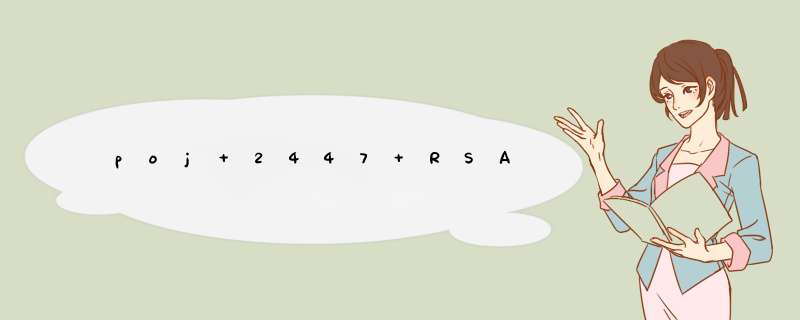
#include <cstdio>#include <cstdlib>#include <ctime>using namespace std;typedef long long LL;#define maxn 10000const int S=20;LL factor[maxn];int tot;LL muti_mod(LL a,LL b,LL c){        a%=c;    b%=c;    LL ret=0;    while (b){        if (b&1){ ret+=a; if (ret>=c) ret-=c;        }        a<<=1;        if (a>=c) a-=c;        b>>=1;    }    return ret;}LL pow_mod(LL x,LL n,LL mod){      if (n==1) return x%mod;    int bit[64],k=0;    while (n){        bit[k++]=n&1;        n>>=1;    }    LL ret=1;    for (k=k-1;k>=0;k--){        ret=muti_mod(ret,ret,mod);        if (bit[k]==1) ret=muti_mod(ret,x,mod);    }    return ret;}bool check(LL a,LL n,LL x,LL t){       LL ret=pow_mod(a,x,n),last=ret;    for (int i=1;i<=t;i++){        ret=muti_mod(ret,ret,n);        if (ret==1&& last!=1&& last!=n-1) return 1;        last=ret;    }    if (ret!=1) return 1;    return 0;}bool Miller_Rabin(LL n){    LL x=n-1,t=0;    while ((x&1)==0) x>>=1,t++;    bool flag=1;    if (t>=1&& (x&1)==1){        for (int k=0;k<S;k++){ LL a=rand()%(n-1)+1; if (check(a,n,x,t)) {flag=1;break;} flag=0;        }    }    if (!flag || n==2) return 0;    return 1;}LL gcd(LL a,LL b){    if (a==0) return 1;    if (a<0) return gcd(-a,b);    while (b){        LL t=a%b; a=b; b=t;    }    return a;}LL Pollard_rho(LL x,LL c){    LL i=1,x0=rand()%x,y=x0,k=2;    while (1){        i++;        x0=(muti_mod(x0,x0,x)+c)%x;        LL d=gcd(y-x0,x);        if (d!=1&& d!=x){ return d;        }        if (y==x0) return x;        if (i==k){ y=x0; k+=k;        }    }}void findfac(LL n){    if (!Miller_Rabin(n)){        factor[tot++] = n;        return;    }    LL p=n;    while (p>=n) p=Pollard_rho(p,rand() % (n-1) +1);    findfac(p);    findfac(n/p);}void gcdExtend(long long a,long long b,long long &d,long long &x,long long &y){     if(!b) {d=a;x=1;y=0;return;}     gcdExtend(b,a%b,d,y,x);     y-=a/b*x;}int main(){    LL C, E, N, T, M, D;    LL x, y, d;    while (~scanf("%lld%lld%lld", &C, &E, &N))    {        tot = 0;        findfac(N);        T = (factor[0] - 1) * (factor[1] - 1);        gcdExtend(E, T, d, x, y);        D = (x % T + T) % T;        M = pow_mod(C, D, N);        printf("%lldn", M);    }    return 0;}