使用scipy.interpole.interpn公司插值N维数组

使用scipy.interpole.interpn公司插值N维数组

我会试着用2D向你解释这件事，这样你就能更好地了解事情的来龙去脉
正在发生。首先，让我们创建一个线性阵列来进行测试。

import numpy as npimport matplotlib.pyplot as pltfrom mpl_toolkits.mplot3d import Axes3Dfrom matplotlib import cm# Set up grid and array of valuesx1 = np.arange(10)x2 = np.arange(10)arr = x1 + x2[:, np.newaxis]# Set up grid for plottingX, Y = np.meshgrid(x1, x2)# Plot the values as a surface plot to depictfig = plt.figure()ax = fig.gca(projection='3d')surf = ax.plot_surface(X, Y, arr, rstride=1, cstride=1, cmap=cm.jet, linewidth=0, alpha=0.8)fig.colorbar(surf, shrink=0.5, aspect=5)

然后，假设你想沿着一条线插值，也就是说，沿着一个点第一维度，但所有点都沿第二维度。这些要点
显然不在原始数组

（x1，x2）

中。假设我们想插值到“x1=3.5”点，该点位于x1轴。

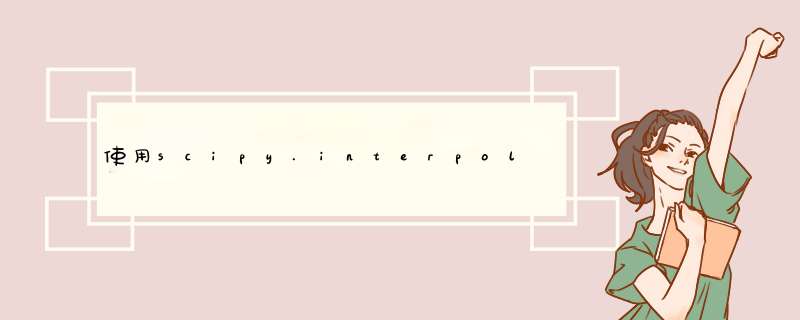
from scipy.interpolate import interpninterp_x = 3.5# only one value on the x1-axisinterp_y = np.arange(10) # A range of values on the x2-axis# Note the following two lines that are used to set up the# interpolation points as a 10x2 array!interp_mesh = np.array(np.meshgrid(interp_x, interp_y))interp_points = np.rollaxis(interp_mesh, 0, 3).reshape((10, 2))# Perform the interpolationinterp_arr = interpn((x1, x2), arr, interp_points)# Plot the resultax.scatter(interp_x * np.ones(interp_y.shape), interp_y, interp_arr, s=20,c='k', depthshade=False)plt.xlabel('x1')plt.ylabel('x2')plt.show()

这会得到所需的结果：请注意，黑点的位置正确
在平面上，x1值为3.5。!【插值曲面图】
[分数](https://i.stack.imgur.com/AKDzK.png)
请注意，大多数的“魔力”，以及你的问题的答案，在于这些
两条线：

interp_mesh = np.array(np.meshgrid(interp_x, interp_y))interp_points = np.rollaxis(interp_mesh, 0, 3).reshape((10, 2))

我已经解释了它的工作原理
其他地方. 总之，这是什么
做的是创建一个大小为10x2的数组，包含10的坐标
要在“arr”处插入的点。（唯一的区别是
我为这篇文章写了一个解释

np.mgrid公司

，其中
是写作的捷径

np.网格

为了一群阿兰奇）
对于您的4x4案例，您可能需要以下内容：

interp_mesh = np.meshgrid([0.1], [9], np.linspace(0, 30, 3),    np.linspace(0, 0.3, 4))interp_points = np.rollaxis(interp_mesh, 0, 5)interp_points = interp_points.reshape((interp_mesh.size // 4, 4))result = interpn(points, arr, interp_points)

Hope that helps!